EX (1.2) P6

EX (1.2) P5

EX (1.2) P4

EX (1.2) P3

EX (1.2) P1

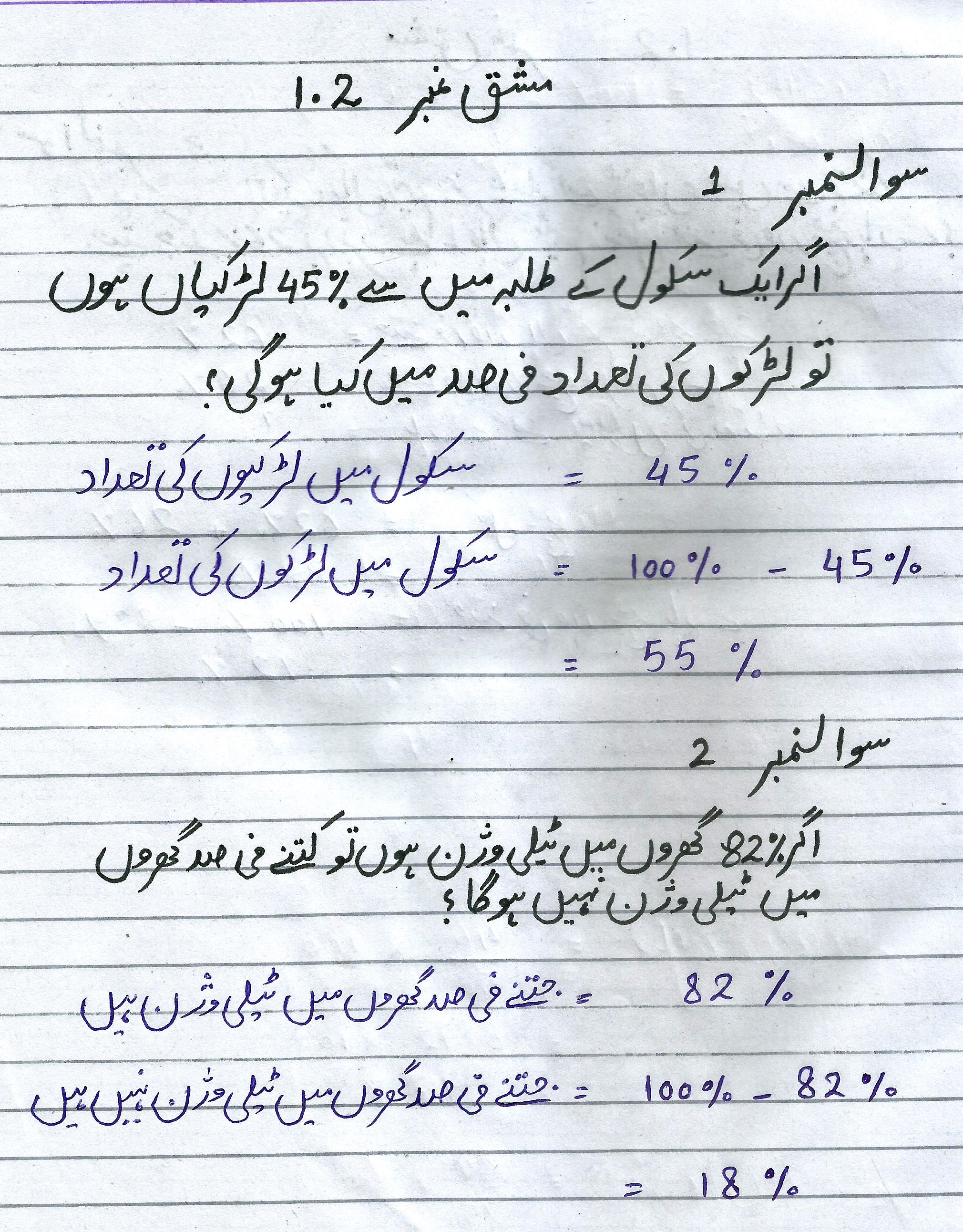
class 10 ex 3 Q2 A

 

Chapter #1
Exercise # 1.3

Q.NO. 2

i. 2/3 : 3/5

=2/3 x15 : 3/5 x15

=30/3 : 45/5

=10 : 9

ii. 4/5 : 3/4

=4/5 x20 : 3/4 x20

=80/5 : 60/4

=16 : 15

iii. 5/6 :7/10

=5/6 x30 : 7/10x30

=150/6 : 210/30

=25 : 21

Class 9 ex3 Q1

 

Chapter # 1

Exerise # 1.3

Q.NO.1

i. Rs. 24,Rs.6

=24:6

Divided by 6

=4:1

ii. 20 kg,5kg

=20:5

Divided by 5

=4:1

iii. 20cm,80cm

=20:80

Divided by 10

=2:8

Divided by 2

=1:4

iv. 5m,5m

=5:5

Divided by 5

=1:1

v. 1500km,1200km

=1500:1200

Divided by 100

`=15:12

Divided by 3

=5:4

vi. Rs.150,Rs.275

=150:275

Divided by 5

=30:55

Divided by 5

=6:11s

Class 7 ex1 Q4

 Chapter no. 1

Exercise # 1.1

Q.NO. 4 Darj zail asharia ko fesad mein tabdeel kijiye

i. 0.5

=0.5x100

=50%

ii. 0.9

=0.9x100

=90%

iii. 1.25

=1.25x100

=125%

iv. 1.39

=1.39x100

=139%

v. 1.72

=1.72x100

=172%

vi. 0.22

=0.22x100

=22%

Class 5 ex1 Q3

 Chapter no. 1

Exercise # 1.1

Q.NO.3 Di gai fesad ko asharia mein tabdeel kijiye jb k jawab teen darga  asharia tak drust ho

i. 47%

=47/100

=0.47

ii. 58%

=58/100

=0.58

iii. 92%

=92/100

=0.92

iv. 8%

=8/100

=0.08

v. 12%

=12/100

=0.12

vi. 120%

=120/100

=1.2

Class 4 ex1 Q2

 Chapter no. 1

Exercise # 1.1

Q.NO.2 Darjzail kasoor ko fesad ki shakal mein likhiye 

vii. 23/60

=23x100/60

=2300/60%

=38.3%

viii. 8/3

=8x100/3

=800/3%

=266.7%

ix. 8/5

=8x100/5

=800/5%

=160%

x. 7/8

=7x100/8

=700/8%

=87.5%

xi. 5/8

=5x100/8

=500/8%

=62.5%

xii. 3/8

=3x100/8

=300/8%

=37.5%

Class 3 ex1 Q2

 Chapter no. 1

Exercise # 1.1

Q.NO.2 Darjzail kasoor ko fesad ki shakal mein likhiye 

i. 3/4

=3x100/4

=300/4%

=75%

ii. 3/5

=3x100/5

=300/5%

=60%

iii. 4/25

=4x100/25

=400/25%

=16%

iv. 13/20

=13x100/20

=1300/20%

=65%

v. 31/25

=31x100/25

=3100/25%

=124%

vi. 21/40

=21x100/40

=2100/40%

=52.5%

find the interval satisfying the inequality 4 - (1/2)n < -7 + (1/4)n,

To find the interval satisfying the inequality 4 - (1/2)n < -7 + (1/4)n, we can solve it step by step. Let's begin:

4 - (1/2)n < -7 + (1/4)n

First, let's eliminate the fractions by multiplying the entire inequality by the least common multiple (LCM) of the denominators, which is 4. This gives:

4 * (4 - (1/2)n) < 4 * (-7 + (1/4)n)

Simplifying:

16 - 2n < -28 + n

Now, let's isolate the variable n on one side of the inequality. We can do this by adding 2n to both sides and adding 28 to both sides:

16 - 2n + 2n < -28 + n + 2n + 28

16 < 3n

Finally, divide both sides of the inequality by 3:

16/3 < n

So, the solution to the inequality is n > 16/3.

Therefore, the interval satisfying the inequality is (16/3, ∞), where n is greater than 16/3.

by using methmatical induction , prove that the eqvation 2+4+6+....2n =n(n+1) is true for all positive integers

 To prove the equation 2 + 4 + 6 + ... + 2n = n(n + 1) for all positive integers using mathematical induction, we need to show that it holds for the base case (n = 1) and then demonstrate that if it holds for some arbitrary positive integer k, it also holds for k + 1.

**Step 1: Base Case**

Let's check the equation for the base case when n = 1:

2 = 1(1 + 1)

2 = 1(2)

2 = 2

The equation holds true for the base case.

**Step 2: Inductive Hypothesis**

Assume that the equation holds true for some arbitrary positive integer k, that is, assume that:

2 + 4 + 6 + ... + 2k = k(k + 1)

**Step 3: Inductive Step**

We need to show that if the equation holds for k, it also holds for k + 1.

We start with the left-hand side (LHS) of the equation:

2 + 4 + 6 + ... + 2k + 2(k + 1)

Using the assumption from the inductive hypothesis:

= k(k + 1) + 2(k + 1)

Factoring out (k + 1):

= (k + 1)(k + 2)

This matches the right-hand side (RHS) of the equation, which is n(n + 1) when n = k + 1:

= (k + 1)((k + 1) + 1)

= (k + 1)(k + 2)

Therefore, if the equation holds for k, it also holds for k + 1.

**Step 4: Conclusion**

By proving the base case and showing that if it holds for k, it also holds for k + 1, we have established that the equation 2 + 4 + 6 + ... + 2n = n(n + 1) is true for all positive integers by mathematical induction.

Physics SEM2

 Q2 )

Explain the motion of an object in the following situation which suitable example A speed is constant and velocity is varying B acceleration of a body is constant but velocity is zero C speed is constant and acceleration is zero D force is applied but acceleration is zero

Solution

A) Speed is constant, and velocity is changing:

In this scenario, the object's speed remains the same, but the direction of its velocity is changing. This situation occurs when an object moves in a circular path at a constant speed. For example, consider a car driving in a circular track at a constant speed of 60 miles per hour. Although the car maintains the same speed, its velocity is constantly changing because it keeps changing direction as it moves along the circular path.

B) Acceleration of a body is constant, but velocity is zero:

In this case, the object is not moving (velocity is zero), but it experiences a constant acceleration. An example of this is an object in free fall. Imagine dropping a ball from a height. At the moment it is released, its velocity is zero, but it accelerates downward due to the force of gravity. During its descent, the ball's velocity remains zero, but its acceleration is constant (approximately 9.8 m/s² downward).

C) Speed is constant, and acceleration is zero:

When an object maintains a constant speed and its acceleration is zero, it means that there is no change in its velocity over time. An example could be a car traveling on a straight road at a constant speed of 50 miles per hour. As long as there are no external forces or changes in conditions, the car will continue to move at the same speed in a straight line without any change in its velocity.

D) Force is applied, but acceleration is zero:

In this scenario, a force is being applied to an object, but its acceleration is zero. This situation occurs when the applied force is balanced by an equal and opposite force, resulting in a state of equilibrium. A common example is a book resting on a table. The force of gravity pulls the book downward, but the table exerts an equal and opposite force, balancing the gravitational force. As a result, the book remains stationary, and its acceleration is zero.

It's important to note that velocity and acceleration are vector quantities, meaning they have both magnitude and direction. Speed, on the other hand, is a scalar quantity that only represents the magnitude of velocity.

Physics SEM 1

 Q 1 )

The required take off speed of a commercial airplane is 12 North if dis commercial airplane take 30 seconds to covers the airport Runway Park calculate acceleration required during takeoff and runway length of airport

Solution

To calculate the acceleration required during takeoff, we need to use the formula:

acceleration = (final velocity - initial velocity) / time

Given:

Initial velocity (V0) = 0 m/s (assuming the airplane starts from rest)

Final velocity (V) = 12 m/s

Time (t) = 30 seconds

Plugging in the values into the formula, we have:

acceleration = (12 m/s - 0 m/s) / 30 s

acceleration = 12 m/s / 30 s

acceleration = 0.4 m/s²

Therefore, the acceleration required during takeoff is 0.4 m/s².

To calculate the runway length of the airport, we can use the kinematic equation:

distance = (initial velocity * time) + (0.5 * acceleration * time²)

Plugging in the values, we have:

distance = (0 m/s * 30 s) + (0.5 * 0.4 m/s² * (30 s)²)

distance = 0 m + (0.5 * 0.4 m/s² * 900 s²)

distance = 0 m + (0.2 m/s² * 900 s²)

distance = 0 m + 180 m

distance = 180 m

Therefore, the runway length of the airport is 180 meters.